A Brief History of Zero
Kristen McQuillin, July 1997 (revised January 2004)
Once upon a time there was no zero. Of course people knew if they had nothing, but there was no mathematical notation for it. Zero was independently invented only three times.
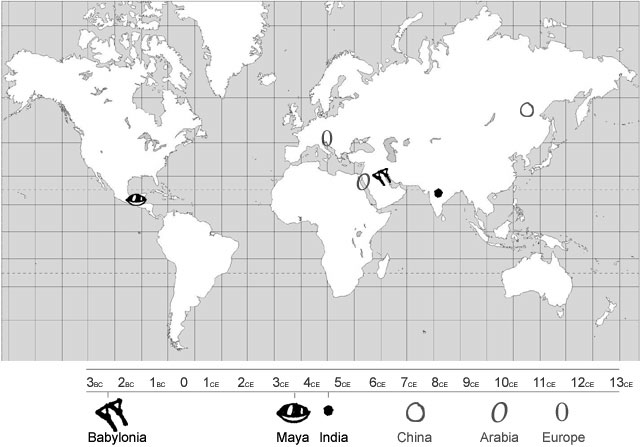
The first recorded zero is attributed to the Babylonians in the 3rd century BC. A long period followed when no one else used a zero place holder. But then the Mayans, halfway around the world in Central America, independently invented zero in the fourth century CE. The final independent invention of zero in India was long debated by scholars, but seems to be set around the middle of the fifth century. It spread to Cambodia around the end of the 7th century. From India it moved into China and then to the Islamic countries. Zero finally reached western Europe in the 12th century.
Before you continue reading the history of zero, please be sure you understand these underlying concepts : Number vs Numeral; Invent vs Discover & Place Value Notation
Babylonia: 300 B.C.
The Babylonians were the first culture to invent the place value system. They had a sexigesimal number system, that is, they counted in 60s, as we count in tens. When you count minutes in an hour or measure circles you are thinking in sexigesimal.
Of course the Babylonians didn’t use our numerals. They wrote in cuneiform, a writing system optimized for writing in damp clay tablets. They used two symbols to represent all the numbers from 1 to 59. The wedge was used for a one and the crescent equalled a 10. By grouping them together, they created symbols for all 59 numbers.

5 crescents + 4 wedges = 54
Beginning at 60, we see a place value. The number 61 would be written with one wedge to the left (1 sixty), and one to the right (1 one).

The number 124 (2 sixties + 4 ones)
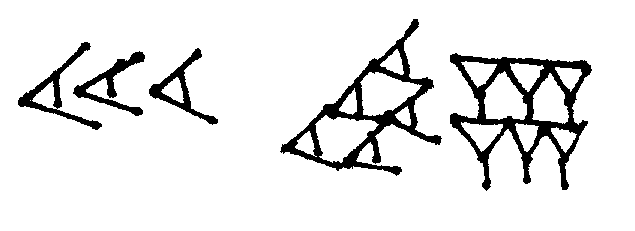
The number 1856 (30 sixties + 56 ones)
And here’s the challenge that leads to the invention of zero. How do you indicate that there’s nothing in a particular place? How would you show the number 3604? 3604 is 1 "60 squared" + 4 ones but nothing in the sixties column. Well, scribes started leaving a blank space. But not all of them did that and even when they did sometimes it was a pretty small space—it was difficult to tell it was there. So one very bright scribe put in a symbol that already existed as a separator in literature, a sort of sideways, superscript, double wedge. Now it was easy to distinguish whether you meant 3604 or 64:
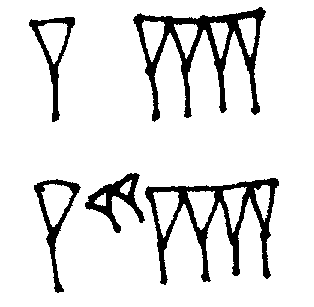
top: 64 (1 sixty + 4 ones)
bottom: 3604 (1 sixty2 + 0 sixty + 4 ones)
Babylonian mathematicians used the separator (effectively the first zero) in the middle position only. The person doing the calculations knew what order of magnitude he was working with and didn’t add any separators at the end of his notations. However, the astronomers started using the zero placeholder in at the end and at the beginning of notations. This allowed them to note fractional degrees and minutes of arc and made their computations more accurate.
Despite the invention of zero as a placeholder, the Babylonians never quite discovered zero as a number. On an accounting tablet recording the distribution of grain there is a notation at the end of a column of numbers that reads “The grain is exhausted.” Another example from the same era is a description subtracting 20 from 20: “twenty minus twenty…you see.”
Although we have evidence of zero from tablets in the Selucid era (4th to 1st C BC), it is possible that the zero was invented before that time. Many of the Seleucid era tablets are copies of much older documents. We’ll never know for certain, so we place the Babylonia zero around the 3rd century BC.
Central America: 350 CE
 The Mayans, native inhabitants of
Central America, were highly skilled mathematicians, astronomers, artists
and architects. However, they failed to make other key discoveries and inventions
that might have helped their culture survive. They never used the plow or
metal tools and their civilization collapsed mysteriously around 900 CE.
The Mayans, native inhabitants of
Central America, were highly skilled mathematicians, astronomers, artists
and architects. However, they failed to make other key discoveries and inventions
that might have helped their culture survive. They never used the plow or
metal tools and their civilization collapsed mysteriously around 900 CE.
They had a very complex calendar system and needed a placeholder in their elaborate date system. This lead to their invention of zero—600 years and 12,000 miles removed from the Babylonians.
The Mayans had several calendars. There was a 365 day civil year, a 260 day religious year and, key to their invention of zero, the complicated Long Count calendar which measured time from the start of the Mayan civilization (August 12, 3113 B.C.) and completes a full cycle on December 21, 2012.
Mayan Long Count Units | ||
|---|---|---|
kin |
day |
|
unial |
month |
20 days |
tun |
year |
360 days (18 months) |
katun |
20 tuns |
7200 days (20 years) |
baktun |
20 katuns |
144,000 days (400 years) |
pictun |
20 baktuns |
2,880,000 days (8,000 years) |
calabtun |
20 pictuns |
57,600,000 days (160,000 years) |
kinchiltun |
20 calabtuns |
1,152,000,000 days (3,200,000 years) |
alautun |
20 kinchiltuns |
23,040,000,000 days (64 million years) |
It is the formal Long Count calendar that brought about the zero. The Mayan numerals were very complex in formal use—painted or carved heads or even full figures were used to represent numbers. When using these ornate carvings on a stelae, or stone tablet, the Mayans had a rather rigid graphic layout; each period of time had a space and all the spaces needed to be filled in. So a date that was 8 baktuns, 14 katuns, 3 tuns, 0 unials and 12 kins had to have one figure for each place. The zero was often represented by a shell shape.
Despite the use of zero in the place value system, it was never used for calculations. Once again, this stems back to the calendar. You may have noticed in the chart above that a 360 day year is 18 months (20 days to a month). This irregularity messed up an otherwise tidy vegisimal (base 20) system:
Decimal 10 is (1 x 10) + (0 x 1) = 10
Vegisimal 10 is (1 x 20) + (0 x 1) = 20
Mayan 10 is (1 x 20) + (0 x 1) = 20
Decimal 100 is (1 x 10exp2) + (0 x 10) + (0 x 1) = 100
Vegisimal 100 is (1 x 20exp2) + (0 x 20) + (0 x 1) = 400
Mayan 100 is (1 x (18x20)) + (0 x 20) + (0 x 1) = 360
Decimal 1000 is (1 x 10exp3) + (0 x 10exp2) + (0 x 10) + (0 x 1) = 1000
Vegisimal 1000 is (1 x 20exp3) + (0 x 20exp2) + (0 x 20) + (0 x 1) = 8000
Mayan 1000 is (1 x (18x20exp2)) + (0 x (18x20)) + (0 x 20) + (0
x 1) = 7200
India: 458 A.D. (debated)
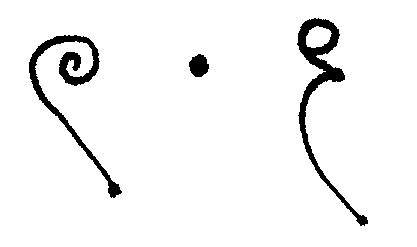 The final independent invention of
the zero was in India. However, the time and the independence of this invention
has been debated. Some say that Babylonian astronomy, with its zero, was
passed on to Hindu astronomers but there is no absolute proof of this, so
most scholars give the Hindus credit for coming up with zero on their own.
The final independent invention of
the zero was in India. However, the time and the independence of this invention
has been debated. Some say that Babylonian astronomy, with its zero, was
passed on to Hindu astronomers but there is no absolute proof of this, so
most scholars give the Hindus credit for coming up with zero on their own.
The reason the date of the Hindu zero is in question is because of how it came to be.
Most existing ancient Indian mathematical texts are really copies that are at most a few hundred years old. And these copies are copies of copies of copies passed through the ages. But the transcriptions are error free…can you imagine copying a math book without making any errors? Were the Hindus very good proofreaders? They had a trick.
Math problems were written in verse and could be easily memorised, chanted, or sung. Each word in the verse corresponded to a number. For example,
viya dambar akasasa sunya yama rama veda
sky (0) atmosphere (0) space (0) void (0) primordial couple (2) Rama (3)
Veda (4)
0 0 0 0 2 3 4
Indian place notation moved from left to right with ones place coming first.
So the phrase above translates to 4,230,000. 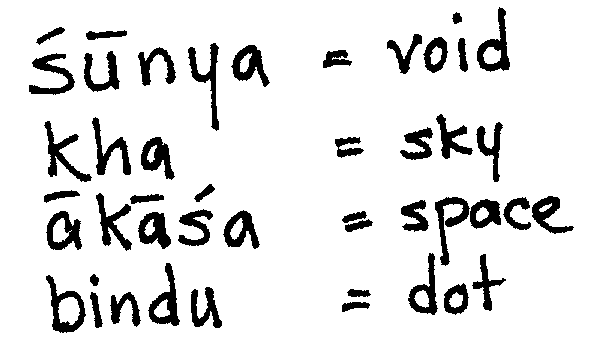
Using a vocabulary of symbolic words to note zero is known from the 458 AD cosmology text Lokavibhaga. But as a more traditional numeral—a dot or an open circle—there is no record until 628, though it is recorded as if well-understood at that time so it’s likely zero as a symbol was used before 628.
Which it probably was, considering that 30 years previously, an inscription of a date using a zero symbol in the Hindu manner was made in Cambodia.
A striking note about the Hindu zero is that, unlike the Babylonian and Mayan zero, the Hindu zero symbol came to be understood as meaning “nothing.” This is probably because of the use of number words that preceded the symbolic zero.
Spreading Outward: China, Arabia and Europe
The Hindus influenced the numeration of nearby locales, and introduced the zero to the Chinese and to the Arabs who developed the modern day shape of numerals and passed them, along with zero, to the Europeans in the 12th century.
Although China independently invented place value, they didn’t make the leap to zero until it was introduced to them by a Buddhist astronomer (by way of India) in 718.
Although it seems strange to image a place value system with no place holder for “nothing,” it makes perfect sense when you see the Chinese method for writing and calculating numbers.
The Chinese used a counting board to do their mat, and an additive system to write their numbers. There was a symbol for 1 and a symbol for five and these symbols were added together to form symbols for other numbers up to 9. The numbers were actually rods arranged on a counting board which ran from left to right. Any missing places were left blank on the counting board. After the introduction of the zero symbol, the counting board could be retired. Numbers could be written on paper without the need of little rods and counting boards.

Arab countries in the MIddle East also got their zero from Indian scholars. Arab mathemeticians created a new form of writing numbers—the Arabic numberals we still use today. When Europe and the MIddle East began trade on a large scale, Europe adopted Arabic numerals and abandoned counting boards.
Underlying Concepts
"It must have required many ages to discover that a brace of pheasants and a couple of days were in both instances the number two."
Bertrand Russell
Number vs. Numeral
A number is a quantity, an abstraction of a collection of things; a numeral is a man-made symbol that represents the number.
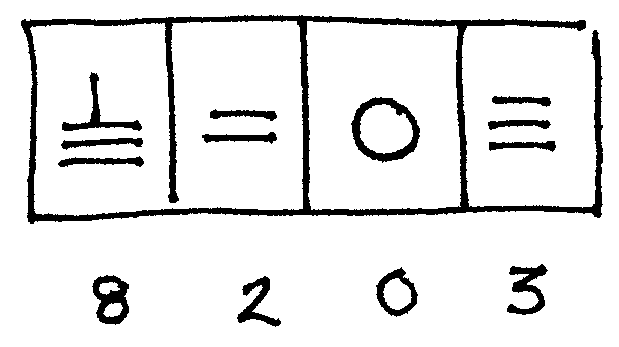
 Numerals (symbols)
from various cultures look different, but all express the same number. Some
are very complex, others are simple dots or circles. All four of these are
symbols for the number zero. L to R they are from Babylonia, China, India
and Central America. Words are also symbols that express numbers, but we
don’t call them numerals.
Numerals (symbols)
from various cultures look different, but all express the same number. Some
are very complex, others are simple dots or circles. All four of these are
symbols for the number zero. L to R they are from Babylonia, China, India
and Central America. Words are also symbols that express numbers, but we
don’t call them numerals.
Numbers (quantity) are always the same value, no matter what symbol or word is used to represent them. Uno, bindu, ichi, 1, single, solitary. All of these symbols represent the concept we know as “one.” The quanity does not change, even when the symbol is different.
Zero is a special case. Constance Reid, in From Zero to Infinity shows the difference between number and numeral with a set of simple math problems. It is easy to use zero when it is a symbol, but not always so easy to calculate with zero the number. What are the answers to the following math problems?
Zero as Symbol |
Zero as Number |
1 + 10 = |
1 + 0 = |
10 - 1 = |
0 - 1 = |
1 x 10 = |
1 x 0 = |
10 / 1 = |
0 / 1= |
[Answers. symbol column: 11, 9, 10, 10. number column: 1, -1, 0, 0]
Invent vs. Discover
invent (v.) think up or mentally fabricate, esp a new device or
contrivance. The numeral zero (symbol) was invented.
discover (v.) to be the first to find out, see or know about; to
realize. The number zero (abstract) was discovered.
Place Value Notation
Place value notation uses numerals in different positions to represent different numbers. You may recall learning about the “tens” place and the “ones” place in elementary school; this is place value notation.
Our system uses place value notation; for example, 32 means “three tens and two ones.”
|
Tens |
Ones |
3 |
2 |
Place value notation is how zero was invented. It was a symbolic placeholder for an empty place (for example 302 or 3200). Previous to the invention of zero, either number might have been written as 32, leaving the reader to figure out the number from context. Zero was a big improvement for accurate accounting!
In most systems that use place value notation, the places are the exponents of the base. In our decimal (base 10) system we have places for: 1, 10, 10 squared (100), 10 cubed (1000) and so on. In a sexigesimal (base 60) system, the places are 1, 60, 60 squared (3600), etc. In a base 20 system: 1, 20, 20 squared (400), 20 cubed (800), and so on.
Value "Four hundred and twelve" in Base 10 & Base 60
DECIMAL |
10 squared place |
Tens place |
Ones place |
| 4 | 1 | 2 | |
SEXIGESIMAL |
60 squared place |
Sixties place |
Ones place |
| 6 | 52 |
Numerals 4-1-2 in Place Value Notation
DECIMAL |
10 squared place |
Tens place |
Ones place |
| 4 | 1 | 2 | |
SEXIGESIMAL |
60 squared place |
Sixties place |
Ones place |
| 4 | 1 | 2 |
